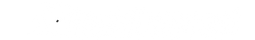Did you solve it? Puzzles for blockheads

Earlier today I set you these five puzzles from Mathigon’s advent calendar. Here they are again, with solutions. Sorry if you came here to read about Ian Dury, but in recompense you get to get your head around these blocks:

1. Hit me with your four cube stick

Solution

2. Timelines

Solution 22 times in 24 hours

If we consider 6:00 AM as the first time, the hands will form a straight line every 1 hour, 5 minutes, and 27 seconds.

3. Eight heavy adult females

Solution 888+88+8+8+8 = 1000

I also asked about how to make 100. That’s 88 + 8 + ((8 + 8 + 8 + 8)/8)

Or 10. That’s 8 + ((8 + 8)/8) + 8 + 8 – 8 – 8

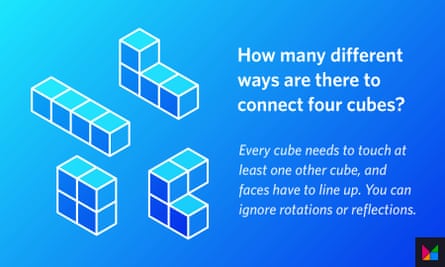
4. Slice of Orange

Solution π/6

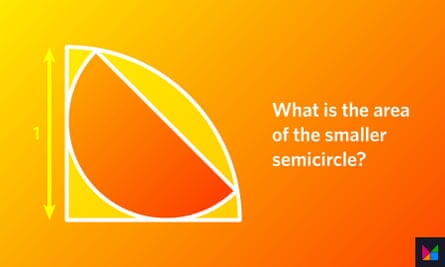
Let C be the centre of the semicircle, and let r be the radius of the semicircle. We can make the right-angled triangle (shaded yellow) OCB that has two sides of length r. Using Pythagoras, the hypotenuse of this triangle has length r√2. Now we use Pythagoras on the red triangle OCE, which has hypotenuse of 1, since this is simple a radius of the large circle. If (r√2)² + r² = 1² then 3r² = 1, or r = 1/√3.

The area of a circle of radius r is πr², so the area of a semicircle of radius r is πr²/2.

The answer is π(1/√3)²/2 = π/(3×2) = π/6

5. Bucket list

Solution 120/286, or about 42 per cent

Let’s enumerate all possible equally likely combinations. First, when all 5 balls go in the same bucket. There are five combinations: (5, 0, 0, 0, 0), (0, 5, 0, 0, 0), and so on. .

Now, let’s consider the ways to place 4 marbles in one bucket, and 1 marble in another, such as (4, 1, 0, 0, 0). For each of the five possible positions of the 4-marble bucket, there are four positions for the 1-marble bucket. So there are 5 x 4 = 20 of this combination.

Carrying on, there are 80 ways to have 3 marbles in one bucket. 20 of these are keeping 3 in one bucket and 2 in another, i.e (3, 2, 0, 0, 0). The logic is the same as 4 and 1 above. And 60 of these are keeping 3 marbles in one bucket and having the other 2 in different buckets. i.e (3, 1, 1, 0, 0). The first solo marble can go in 4 buckets and 2nd solo marble can go in 3 buckets. So there are 5 * 4 * 3 = 60 ways.

There are 5*4*3*2 (120) ways to have 2 in 1 bucket and then the other three in individual buckets. Note – this is the only way with one bucket empty! There are 5*4*3 (60) ways to have 2 in one bucket, 2 in another, and 1 in another. So, there are 180 combinations in this category, with 120 of them being our desired outcome.

There is 1 way to place 1 marble in each bucket.

There are thus 5 + 20 + 80 + 180 + 1 = 286 total combinations, only 120 of them have exactly 1 bucket that remains empty. So the probability is 120/286, or about 42%.

Thanks to Mathigon for today’s puzzles, which appear in their forthcoming Mathigon advent calendar. Mathigon is a free website about maths, with fantastic interactive features. Highly recommended!

I set a puzzle here every two weeks on a Monday. I’m always on the look-out for great puzzles. If you would like to suggest one, email me.

I give school talks about maths and puzzles (online and in person). If your school is interested please get in touch.